When a driver encounters an emergency, he needs to stop the car in the shortest distance possible.
The distance the car travels before coming to a stop after the driver realizes he needs to stop is called stopping distance.
Example 1
The graph shows the minimum stopping distance for a car travelling at different speeds on a dry road.
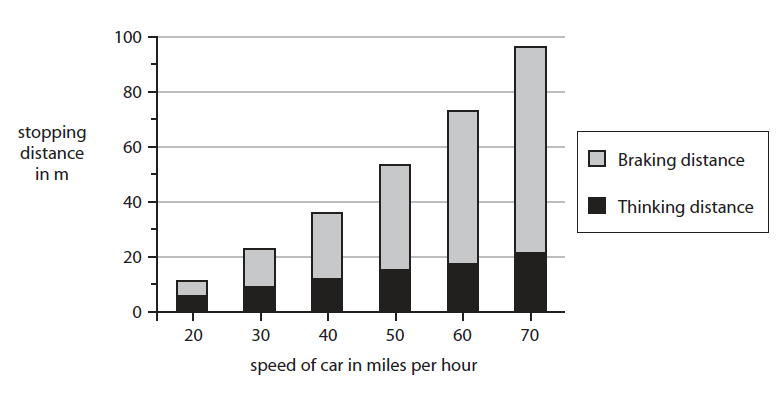
(a) Write an equation to show the link between stopping distance, braking distance and thinking distance.
stopping distance = thinking distance + braking distance
* thinking distance = distance traveled during the time it took for the driver to actually apply the brake after realizing he needs to stop the car
* braking distance = distance traveled after the driver applies the brake
(b) Describe the patterns shown in the graph.
- As the speed of car increases, the braking distance and thinking distance and stopping distance all increase.
- The braking distance increases more than the thinking distance as the speed of car increases.
(c) If the road is icy, describe what change there would be to the thinking distance and the braking distance.
- Since the thinking distance depends on the speed of the car and the reaction time of the driver, there will be no change to the thinking distance.
- There is less friction on ice, so the braking distance will increase.
Example 2
A rabbit runs across the road in front of a car. The driver applies the brakes. State factors that affect the chance of the rabbit escaping without being hit.
- Driver's reaction time, which may be affected by drinking, sleepiness, tiredness and how much attention he was paying to the road
- Road surface conditions, such as dry road, icy road and muddy road
- The force applied to the brake
- Tire conditions
- Distance of rabbit from the car
- Speed of rabbit
- Speed of car
- Mass of car

