·
Trigonometric Identities
In IB Mathematics, there are trigonometric identities as follows.
- sec x = 1/cos x
- cosec x = 1/sin x
- cot x = 1/tan x
- tanθ = sinθ/ cosθ
- cos2θ + sin2θ = 1
- 1 + tan2θ = sec2θ
- cot2θ + 1 = cosec2θ
Note: When asked to show by counter-example that a statement is false, sub a value in and show that LHS is not equal to RHS.
Formulae for sin (A + B), cos (A + B), tan (A + B)
Sin (A + B) = sin A cos B + cos A sin B
Sin (A - B) = sin A cos B - cos A sin B
Cos (A + B) = cos A cos B - sin A sin B
Cos (A - B) = cos A cos B + sin A sin B
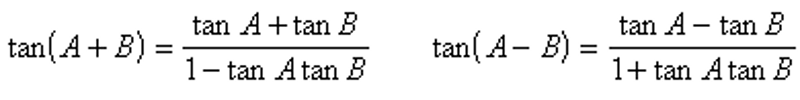
Transformations of Graphs
When it comes to IB Mathematics, the transformations so far follow these rules:
- f(x) + a is f(x) shifted upward a units
- f(x) – a is f(x) shifted downward a units
- f(x + a) is f(x) shifted left a units
- f(x – a) is f(x) shifted right a units
- –f(x) is f(x) flipped upside down ("reflected about the x-axis")
- f(–x) is the mirror of f(x) ("reflected about the y-axis")
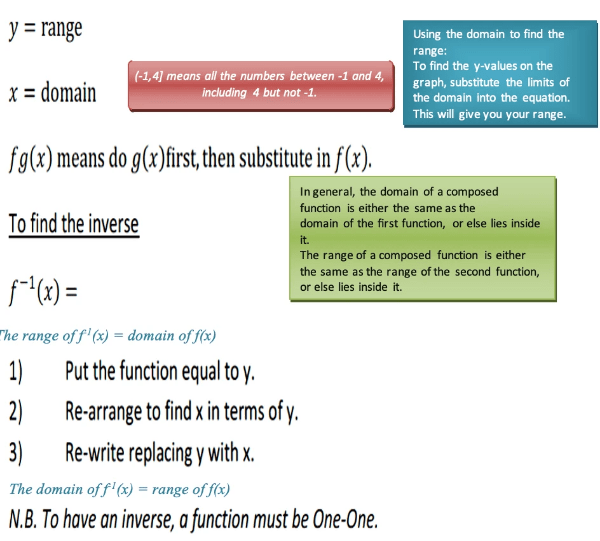
Functions
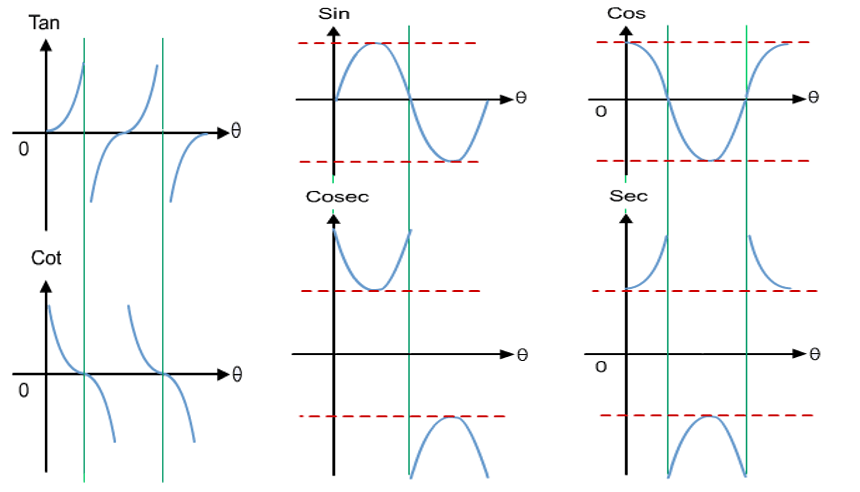
Graphs of Functions
This is the end of this topic.


