Equilibrium Constants
- Consider the reversible reaction A + 3B ⇌ C + 2D
- The rate expression for the forward reaction can be written:
Rate = kf[A][B]3, where kf is the rate constant for the forward reaction.
- The rate expression for the reverse reaction can be written:
Rate = kr[C][D]2, where kr is the rate constant for the reverse reaction.
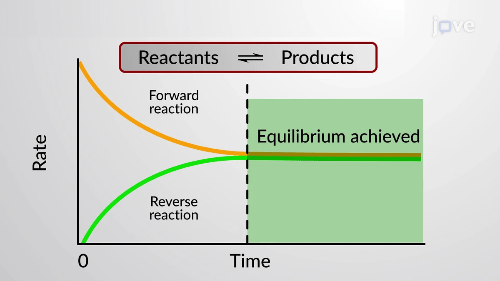
- At equilibrium, the rates of the forward and reverse reactions are equal:
kf[A][B]3 = kr[C][D]2
- This can be rearranged to give kf/ kr = ([C][D]2)/([A][B]3)
- Since the rate constants are constant at a given temperature, it follows that the term
- kf/ kr is also constant at a given temperature. It is therefore normally regarded as a single constant Kc and is known as the equilibrium constant of the reaction.
- Kc = ([C][D]2)/([A][B]3)
Units for equilibrium constant (Kc)
The units of the equilibrium constant vary, depending on the number of species involved. The units can be deduced by multiplying out the units of concentration and cancelling as appropriate. The units of the above equilibrium constant are mol-1dm3.
Eg: H2(g) + I2(g)2HI(g)
Kc = [HI]2 It has no units.
[H2][I2]
Eg: PCl5(g) PCl3(g) + Cl2(g)
Kc = [PCl3][Cl2] It has units of moldm-3.
[PCl5]
Calculation of equilibrium constants (Kc)
If the concentrations of all the reacting species are known, the equilibrium constant can be calculated by putting the values into the expression for the equilibrium constant and computing the value.
Often, however, the concentration of only one of the species at equilibrium is given, and the others must be deduced. This is done by considering the way in which the species react:
Eg: Consider the equilibrium H2(g) + I2(g) ⇌ 2HI(g)
If the initial amounts of hydrogen and iodine are a and b, and x moles of hydrogen react with x moles of iodine to give 2x moles of hydrogen iodide, then the amounts of hydrogen and iodine in the mixture at equilibrium are (a-x) and (b-x) respectively.
H2(g) + I2(g) ⇌2HI(g)
Initially: a b 0
At equilibrium: (a-x) (b-x) 2x
If a, b and the amount of one of the species present at equilibrium is known, then the value of x can be deduced and the amounts of all the other species at equilibrium can also be deduced.
Eg. If 1.5 mol of hydrogen and 1.2 mole of iodine are mixed together and allowed to reach equilibrium, the amount of hydogen iodide present at equilibrium is found to be 1.6 mol.
2x = 1.6, so x = 0.8, (a-x) = 0.7 and (b-x) = 0.4
Therefore at equilibrium there are 0.7 moles of hydrogen, 0.4 moles of iodine and 1.6 moles of hydrogen iodide.
These amounts can be converted into concentrations, and then inserted into the expression for equilibrium constant.
These types of question often come up in AS/A-level Chemistry, practice makes perfect, you can do it!! 🙌🏻
This is the end of the topic!

Drafted by Cherry (Chemistry)

