UKAT Question Analysis - Quantitative Reasoning Question 13
A horse riding school charges an annual fee per person, on top of which tickets can be purchased to ride. Tickets are sold by the unit, by packs of 5 and packs of 10. One ticket gives the right to one ride. You should assume that users of the school will always choose the purchase method that is the cheapest for them and that they use all the tickets they purchase in the same year as they are purchased.
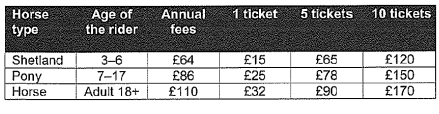
Q13.1 Kathy, 10 years old, bought 45 tickets this year. How much did she spend in total at the school for the year?
a. £86.00
b. £723.00
c. £764.00
d. £788.00
e. £1,211.00
Q13.2 Anna's total cost for one year was £710. She is 30 years old. How many lessors did she take?
a.31
b.32
c.33
d.34
e.35
Q13.3 What discount is the riding school giving on Shetland lessons for those who purchase a block of 5 tickets instead of 5 individual tick-ets?
a. 13.3%
b. 15.4%
c. 18.3%
d. 19.6%
e. 37.6%
Q13.4 The parents of two children aged 9 and 5 respectively have a budget of £960. They wish to offer the two children the same num-ber of lessons each, opting to purchase 10-ticket packs only. How many lessons will they purchase for each child?
a.3
b.10
c.20
d.30
e. 60
Answer and Explanation
Q13.1 — c: £764
Kathy will have spent 86 + (4 x 150) + 78 = £764.
Q13.2 — e: 35
If we deduct the annual fee from the total fee, we have 710 — 110 = £600 spent on actual tickets. We know from the range of the answers that she will have bought 31 to 35 tickets and, therefore, she will have bought at least 3 blocks of 10 tickets. The cost for those is 170 x 3 = £510.
This leaves 600 — 510 = 90 to purchase the remaining tickets, which hap-pens to be the cost for a block of 5 tickets. Therefore she purchased a total of 35 tickets.
Q13.3 - a: 13.3%
Purchasing 5 tickets individually would cost 5 x 15 = £75, so there is a sav-ing of £10 on the £65 cost of a 5-ticket block. The discount is therefore 10 / 75 = 0.133, i.e. 13.3%.
Q13.4 - d: 30
The annual fee for both children is 64 + 86 = £150, leaving 960 — 150 = £810 to purchase actual lessons. Purchasing 10 lessons for each child will cost 120 + 150 = £270. Therefore each child will have 810 / 270 = 3 lots of 10 lessons, i.e. 30 lessons.
Drafted by Juno(UCAT Prep)

