·
Reciprocals
- Flip the fraction to get the reciprocal.
5 = ⁵⁄1 ; ⁵⁄1 ⟶ ⅕
- For the negative reciprocal, switch the sign and flip the fraction.
-½ ⟶ ²⁄1 ; ²⁄6 ⟶ -⁶⁄2
Surds
- √a x √b = √ab
- C√a x D√b = CD√ab
- √a ÷ √b = √a⁄b
- C√a ÷ D√b = C⁄D√a⁄b
- √a/√b = √a⁄b
- When adding, treat it like algebra, you can only add together the same surd, so change the √’s to like terms.
- Do this by leaving the same irrational number.
Simplifying Surds
- Find 2 numbers that are factors, one must be a square number.
- Simplify ⟶ Root of square number goes outside, other number goes inside.
Expanding surds
- Square a — ²a
- Multiply a² x b
- √a² x √b = √a²b
Simplifying Surd x Surd
- Times outside numbers together
- Times irrational numbers together
- Simplify further, want biggest possible number outside.
- Recognise square factors, root then multiply them to the outside number.
Rationalising
- Getting rid of surd from the denominator.
- For normal rationalising, just multiply the surd by itself.
- For rationalising when there is more than just the surd as the denominator, multiply by the opposite.
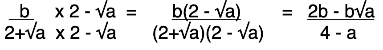
Limits of Accuracy
- Degree of rounding
- E.g. 1100 = 2 s.f.
- Find the values that would round to 1100 for 2sf
- 1050 ≤ 1100 < 1150
- Max speed = greatest distance/shortest time
- Population density = total population/total area
That's the end of the topic!

Drafted by Bonnie (Mathematics)

